
The acceleration is the time rate of change of velocity: a = dv/dt = -g * (1 + v^2 / Vt^2) Multiply the last term by g/g and use the definition of the terminal velocity to obtain: a = -g * (1 + v^2 / Vt^2) Acceleration Notice that the acceleration changes with time. With the positive vertical coordinate denoted by y, the net vertical force Fnet acting on the ball is given by: Fnet = -W -Dīecause the weight of the object is a constant, we can use the simple form of Newton’s second law to solve for the vertical acceleration: Fnet = m a = -W – D m a = – (m * g) – (.5 * Cd * rho * A * v^2) a = -g – (Cd * rho * A * v^2) / (2 * m)
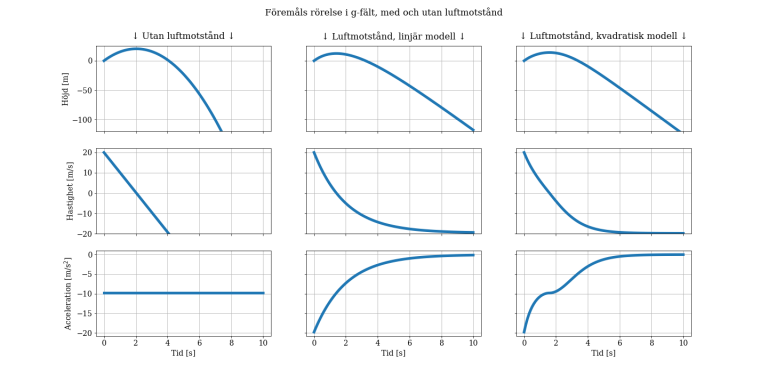
Now, turning to the ascent trajectory, the ball is traveling at an initial vertical velocity Vo. 5 * Cd * rho * A * Vt^2 Vt = sqrt ( (2 * m * g) / (Cd * rho * A) ) Combining the last three equations, we can determine the terminal velocity: m * g =. The gas density has different surface values on the Earth and on Mars and varies with altitude. This is the standard symbol used by aeronautical engineers. On the figure at the top, the density is expressed by the Greek symbol “rho”. Where rho is the gas density, Cd is the drag coefficient which characterizes the effects of shape of the ball, A is the cross-sectional area of the ball, and Vt is the terminal velocity. (The gravitational acceleration has different values on the Moon and on Mars.) The drag is given by the drag equation: D =. Where m is the mass of the object and g is the gravitational acceleration equal to 32.2 ft/sec^2 or 9.8 m/sec^2 on the surface of the Earth. The weight of any object is given by the weight equation: W = m * g Where a is the acceleration, W is the weight, and D is the drag. There is no net force acting on the ball and the vertical acceleration is zero. Vertical Descentĭuring the vertical descent, for a light object, the weight and drag of an object are equal and opposite. Terminal velocity is noted by the symbol Vt. There is a characteristic velocity which appears in many of the equations that is called the terminal velocity because it is the constant velocity that the object sustains during a coasting descent. In the vertical plane, the only forces acting on the ball are the forces of weight and drag. We will first consider the vertical component and then develop the equations for the horizontal component. Unlike the ballistic flight equations, the horizontal equation includes the action of aerodynamic drag on the ball. Vertical LocationĪt launch the ball is inclined at some angle to the vertical, so we resolve the initial velocity into a vertical and horizontal component. On this page we develop the equations which describe the motion of a flying ball including the effects of drag.
In reality, a baseball or a soccer ball in flight generates a moderate amount of aerodynamic drag and is not strictly ballistic. This type of flight is called ballistic flight and assumes that weight is the only force acting on the ball. Home > Beginners Guide to Aeronautics Flight Equations with DragĪ ball in flight has no engine to produce thrust, so the resulting flight is similar to the flight of shell from a cannon, or a bullet from a gun.


 0 kommentar(er)
0 kommentar(er)
